-
Maximal Entropy of the Boltzmann Distribution: A Quantum Perspective
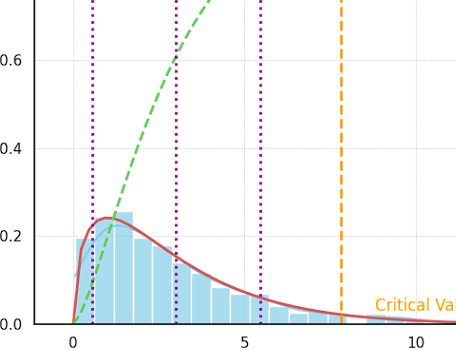
Specifically, in the classical limit (when βEn << 1), the quantum energy levels become very close to each other, and the partition function can be approximated by an integral over continuous energy states rather than a sum over discrete states. In this limit, the quantum Boltzmann distribution approaches the classical result...
-
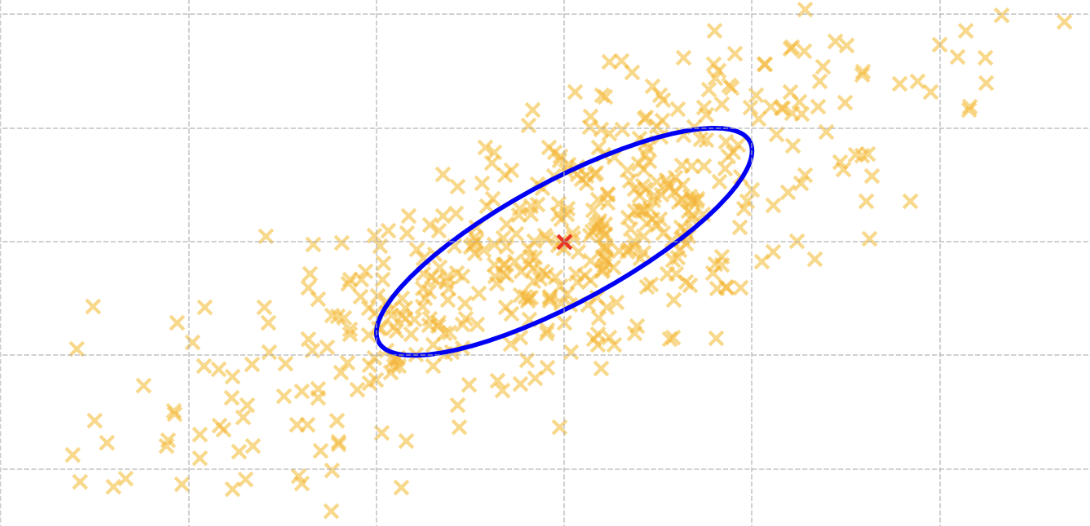
A Sketch of Proofs for Some Properties of Multivariate Gaussian Distribution
When we diagonalize the covariance matrix, we essentially rotate the space such that the axes align with the principal directions of variation...
-
Is the Transition from Univariate to Multivariate Gaussian Distribution Linear?
Now that we have the foundation in place, let’s shift gears and consider the generalization of the univariate Gaussian to higher dimensions. In the multivariate case, we are no longer dealing with a single random variable, but rather a vector of random variables...
-
A Supplementary Discussion on Correlation Coefficients
Yet covariance itself is sensitive to the original units of measurement, limiting its direct interpretability across different data scales...
-
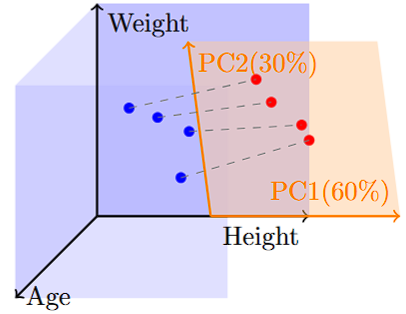
An Introductory Look at Covariance and the Mean Vector
If the mean vector gives us a sense of location, then the covariance matrix gives us a sense of shape...